The secret of the synchronized pendulums clipping
March 30th, 2022
Taken from the January 2020 issue of Physics World.
The fact that pairs of moving pendulums can become synchronized was first observed by the great Dutch scientist Christiaan Huygens back in the 17th century. But as Jonatan Pena Ramirez and Henk Nijmeijer explain, synchronized pendulums still have today’s researchers scratching their heads
Ballet dancers moving in harmony to the rhythm of music. Violinists in an orchestra playing perfectly in unison. A school of fish swimming gracefully together in the sea.
Synchronization – two or more events happening at the same time – is one of the most common phenomena in nature. Extending from unconscious entities to human beings, it’s even an Olympic sport in the form of synchronized swimming or diving. Synchronization is essential to life too. Pacemaker cells, for example, have to fire electrical discharges synchronously to ensure our hearts beat properly.
For physicists, synchronization is particularly intriguing in inert systems. Place two identical metronomes on a wooden bar mounted on two fizzy-drink cans, and you’ll find that the rhythms of these mechanical devices – used by musicians to keep time – can synchronize within minutes or even seconds. But how do the metronomes "decide" to reach a common rhythm? And, more importantly, why do they do this?
To answer those questions, we need to wind back to the 17th century and enter the world of Christiaan Huygens – perhaps the greatest Dutch scientist of all time. Besides having a passion for astronomy and optics, Huygens was also a supreme mathematician. Indeed, his 1673 book Horologium Oscillatorium is one of the most important scientific works of his era, with Isaac Newton believing Huygens to be "the most elegant writer of modern times".
Huygens also made various inventions, including the pendulum clock, which he believed could help to solve one of the greatest scientific challenges of the day. Lacking the location technology we take for granted today, sailors had to determine their latitude by estimating the height of the Sun above the horizon and the angle its rays make with the Earth’s equator. However, they had no physical reference for determining their longitude – how far east or west their ship was at sea.
Most ideas for solving the "longitude problem" consisted of determining the difference between the local time (obtained from the Sun) and the "reference" time at the port from where the ship had set sail. But Huygens wanted to show that his pendulum clocks would be the answer by providing a reliable and accurate reference time to sailors. And so it was that in 1664 Huygens planned a daring experiment with Alexander Bruce, one of the dozen men who had founded the Royal Society in London just a few years before.

A problem of motion
To test their hypothesis, Huygens and Bruce placed two pendulum clocks on board a ship commanded by the British admiral Robert Holmes. The idea of having multiple clocks was that if rough seas stopped one of the clocks from working, at least the other would be running at the correct frequency.
According to the instructions Huygens provided, the ship’s sailors were meant to look at the time indicated by the clocks (set to the time of the place of departure) and then determine the local time (indicated by the Sun). If the time on the clocks was later than the local time, it meant the sailors were moving east. But if the clock time was earlier, they were heading west. In both cases, each hour of difference was equivalent to 15° of longitude.
Departing from the island of St Thomas off the coast of Guinea in west Africa, Holmes’ vessel initially set off in a westerly direction. But after a few days, the master of the ship realized they were running out of drinking water. Using the time indicated by the pendulum clocks, however, Holmes was able to figure out the vessel’s location – barely 30 nautical leagues from one of the Cape Verde islands in the mid-Atlantic. Thanks to this vital information, Holmes steered the vessel towards the island and arrived safely the next day, exactly as expected from the calculations carried out using data from the clocks (1665 Phil. Trans. 1 13).
Based on this successful trial, Huygens – who was as much an entrepreneur as a scientist – drew up a business plan and started to prepare his maritime pendulum clocks for sale. Huygens also published his instructions to determine longitude at sea with these devices (1669 Phil. Trans. 4 937). There was, though, one snag with Huygens’ set-up. It’s almost impossible to build two identical pendulum clocks, which means that one will always be slower or faster than the other. So if one of the clocks were to stop due to rough seas, then even if the sailors reactivated it to match the time of the other clock, it could be substantially "out" and give a wrong longitude. Even an error of just four minutes would lead to a 1° error in the ship’s longitude, which could be disastrous.

Moving in sympathy
While working on the longitude problem, however, Huygens was surprised to discover that if he hung two of his pendulum clocks from a common support – a wooden bar supported by two chairs – the devices kept pace relative to each other. The two pendulums always swung at the same frequency albeit in opposite directions to each other. According to Huygens’ reports, it took the two pendulums about half an hour to reach this out-of-phase synchronization. In a letter to the Belgian mathematician René-François de Sluse, dated 22 February 1665, Huygens referred to this odd phenomenon as "the sympathy of two clocks".
Huygens referred to this odd phenomenon as "the sympathy of two clocks"
For Huygens the beauty of the system was that even if one of the clocks stopped, the other would be keeping the correct time – and hence give the correct longitude measurement. In 1667 he therefore carried out a second maritime experiment, installing two linked clocks on a ship bound for the West Indies. Unfortunately, the clocks came to a stop in a storm and the sailor responsible for them failed to follow the instructions for resetting the devices – making it impossible to measure the time on board with them anymore.
Due to the practical difficulties, Huygens began to realize that pendulum clocks might not be the solution to the longitude problem, at least not in rough seas. Indeed, in December 1683, he sent a letter to the Dutch mathematician Bernard Fullenious, writing that "there is no small hope that it will succeed". In the end, it was the English clockmaker John Harrison who came up with the final solution to the longitude problem almost a century later.
Further progress on what’s now known as "Huygens synchronization" did not occur until 1740 when John Ellicott – a renowned English clockmaker – submitted a manuscript to the Royal Society. In it he described an "odd" phenomenon: when two pendulum clocks were placed next to each other so that the pendulums were oscillating in the same plane, one of them always stopped working after about two hours, whereas the other kept swinging normally. Ellicott concluded that the two pendulum clocks were influencing each other through the common structure on which they were placed.
Little further progress was made on synchronized pendulums until 1873 when the British astronomer and meteorologist William Ellis, while working at the Royal Observatory in Greenwich in 1873, observed two pendulum clocks that had been placed on a wooden stand. Over nine consecutive days, he noticed that the times indicated by the clocks were identical, even though one pendulum was swinging to the left and the other to the right. The pendulums, in other words, were oscillating at the same frequency, but in opposite directions – exactly as in Huygens’ experiment.
Ellis recounted his findings in a paper published in the Monthly Notices of the Royal Astronomical Society (33 480), where he referred to the phenomenon of two out-of-phase pendulums as a "sympathy" despite neither he – nor Ellicott for that matter – mentioning Huygens’ earlier observations. In fact, the first formal attempt to explain the synchronized motion in Huygens’ pendulum clocks was only made in 1906 by the Dutch mathematician Diederik Korteweg and further progress on this mysterious phenomenon did not occur until the Soviet Union in the 1980s. Working at the Mekhanobr Institute in what was then Leningrad, Iliya Izrailevich Blekhman reproduced Huygens’ experiment using two small pendulum clocks placed on a common wall. Blekhman improved Korteweg’s mathematical model by adding terms describing the source of energy that keeps a pendulum clock running.
Remaining mysteries
All these studies have helped to shed light on the secret of self-synchronization in pendulum clocks. Although Christiaan Huygens didn’t have the mathematical tools to explain the strange phenomenon – differential calculus hadn’t been invented in the mid-17th century – we now know that he correctly understood the underlying mechanism. The synchronization is due to the clocks transferring energy to each other via the coupling bar in the form of mechanical vibrations. The clocks start moving exactly out of phase, in other words, when the vibrations exerted by one pendulum clock on the coupling bar are exactly cancelled by the vibrations exerted by the other.
But many questions remain unanswered, more than 350 years after Huygens’ discovery. What, for example, are the "minimal" requirements for self-synchronization? Will any pair of pendulum clocks eventually synchronize – or only certain types? And can you synchronize more than two pendulums? Only since the start of this millennium have scientists started shedding further light on these questions.
In 2002 a team led by Kurt Wiesenfeld at Georgia Tech in the US designed and built a simplified version of Huygens’ experiment using mechanical metronomes instead of pendulum clocks. They concluded that the sympathy observed by Huygens’ in his clocks is largely influenced by the coupling strength, which is the ratio of the total mass of the pendulums to the total mass of the coupling bar from which the clocks are hanging. A large ratio indicates a strong interaction between the pendulums, whereas a small ratio corresponds to a weak interaction. Furthermore, for a small ratio the pendulums synchronize with opposite phase, whereas for a large ratio the pendulums exhibit a "beating death phenomenon", in which one pendulum keeps oscillating whereas the other comes to a stop (Proc. Roy. Soc. A 458 563), as Ellicott had observed.
At about the same time, James Pantaleon from the University of Alaska Anchorage carried out a fascinating experiment consisting of two metronomes placed on a light wooden board sitting on two empty fizzy-drink cans so the whole system could roll. He found that the metronomes reached a common rhythm but oscillated in the same direction – rather than in opposite ways as Huygens and Ellis had seen.
Mystified by this finding, in 2005 we decided to create our own version of Huygens’ experiment at Eindhoven University of Technology. Our set-up consisted of two metronomes mounted on a rigid metal bar suspended from leaf springs (figure 1). Confirming previous findings, we observed at least two types of synchronized motion. If the bar is relatively light, the metronomes start to oscillate synchronously in the same direction. But if the metal bar is heavier than a certain value, they oscillate at the same frequency but in opposite directions, just as Huygens saw. The critical transition mass in our experiment was found to be 2.35 kg.
1 From old to new
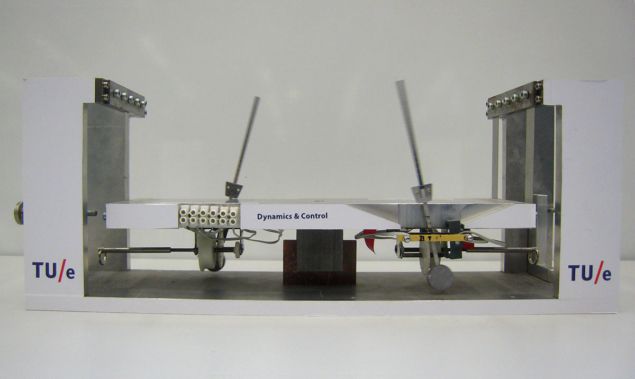
This simplified version of Christiaan Huygens’ original 17th-century experiment on synchronized pendulums was created by the authors at Eindhoven University of Technology in the Netherlands. It consists of two mechanical metronomes coupled through a metallic bar, which is elastically attached to a fixed support by means of springs.
It appears that a light coupling bar facilitates the onset of complete synchronization because the coupling strength is then relatively large. A heavier bar, in contrast, has a weak coupling strength, which results in the metronomes oscillating out of phase. We also derived a mathematical model for our coupled metronomes based on Newton’s second law, which revealed that the key parameters governing the onset of self-synchronization are the metronomes’ mass and oscillation frequency, as well as the mass of the bar and the rigidity of the springs from which the bar is suspended.
Recently, we have reproduced a modern version of Huygens’ experiment (figure 2) using two "monumental" pendulum clocks that were designed and built by Relojes Centenario – a specialist clock-making firm in Zacatlán, Mexico. The pendulum in each clock consists of a 5 kg metal mass attached to the lower end of a wooden rod just under 1 m long. At the heart of each clock is a structure, known as an "anchor-escapement mechanism", that maintains the motion of the pendulum and is responsible for the characteristic "tick-tock" sounds of pendulum clocks. Connected to weights suspended from the clock, this structure gives the pendulum a "kick" each time its angular displacement reaches a certain threshold, thereby regulating the motion of the pendulum as it swings.
2 Monument to history
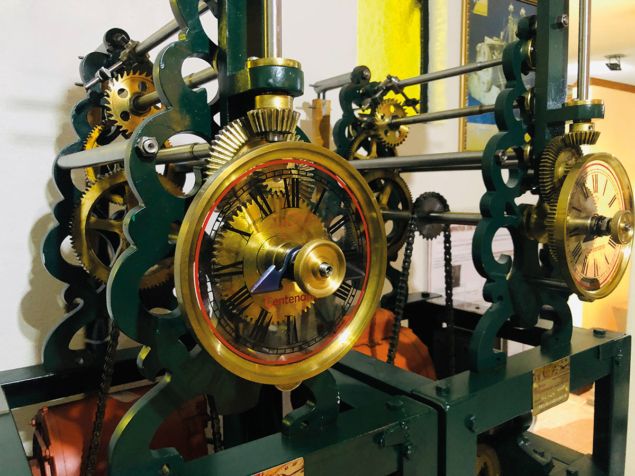
This elaborate modern version of Huygens’ experiment was built by the specialist clock-making company Relojes Centenario in Zacatlán, Puebla, Mexico, and appears in the firm’s museum. Consisting of two pendulum clocks coupled through a wooden structure, the clocks end up moving in complete synchronization. The pendulum in each clock consists of a 5 kg metal mass attached to a wooden rod roughly 1 m long.
Using this equipment, we noticed that, after about 30 minutes, the pendulums were both oscillating in the same direction and at the same frequency. The clocks stayed synchronized for as long as potential energy was stored in the weights to drive the escapement mechanism. Indeed, each clock has a device that rewinds the weights roughly every 30 minutes, which means they could keep running for as long as desired. If it wasn’t in place, the clock would have stopped after about 14 hours.
Using this equipment, we confirmed the secret behind the onset of synchronization – first observed by Huygens all those years ago. As he suspected, it is due to the transmission of vibrations – and thus energy – through the wooden structure on which the clocks are attached. The wooden table, in other words, is the channel by which the pendulums can communicate with each other and eventually "decide" on a common rhythm. Although our modern clocks sat on a pine table, whereas Huygens’ devices hung from a bar, both depend on the elastic deformation of a piece of wood.
The final word
But we have also made some new observations that Huygens never noted. For example, clocks that are placed on the same wooden table and start moving in synchrony are no longer reliable time-keepers, losing 47 seconds per hour, which is almost 19 minutes a day. So even if Huygens had persisted in his use of pendulum clocks to find longitude at sea, and even if those devices weren’t disturbed by stormy seas, they still wouldn’t have worked as good timekeepers (Sci. Rep. 6 23580).
Coupled pendulums can do other strange things too, including "quenching" (both clocks stop working), undergoing seemingly chaotic motion (the clocks oscillate irregularly), moving in an unsynchronized way and more.
So do we now completely understand Huygens’ synchronization? In short, no. Most mathematical models, including our own, aren’t complete in that they don’t consider all the deformations experienced by the wooden support on which the clocks are hanging.
We hope, however, that a complete model will one day be created, not least because Huygens’ system of coupled clocks could shed light on many other systems. Consider for instance two driven "unbalanced" rotors mounted on an elastic support. Under certain conditions, the rotors can revolve synchronously in the same direction but in other cases the rotors start spinning in opposite directions. The latter can be useful as it can cut or even eliminate the vibrations of the common support when the rotors are running. However, synchronized rotation in the same direction is not good as it can make the support vibrate massively, which is bad news if you’ve ever seen or heard a washing machine go out of control.
Studies of Huygens’ clocks could also shed light on the many similar synchronization effects in living organisms. The human body, for example, has many different kinds of oscillating rhythms – including respiration, heartbeat, neuronal activity and blood perfusion – and when these synchronize with each other, very little energy is used. That’s good, but synchronization can also be damaging or dangerous. The generation of epileptic seizures, for example, is closely linked to the (abnormal) synchronization of millions of neurons (see September 2019).
We therefore believe that a thorough investigation of the synchronized pendulum clocks that Huygens first studied all those years ago could help us to get a better understanding of synchronization phenomena throughout the physical and the biological world. Indeed, in one recent paper, we have designed and built a system of synchronized electronic neurons that can – thanks to an "adaptive" training procedure – control a mobile robot driving around an unknown environment while avoiding obstacles (International Journal of Bifurcation and Chaos 26 1650196). Who’d have thought that Huygens’ early observations on his sympathetic clocks would ever lead to such uses?
Keep in touch!